The Official Alexander Sphere Appreciation Page

by Cliff Pickover, Reality Carnival

Mathematicians continue to invent strange objects to test their intuitions. Alexander's horned sphere is an example of a convoluted, intertwined surface for which it is difficult to define an inside and outside. Introduced by mathematician James Waddell Alexander (1888 – 1971), Alexander's horned sphere is formed by successively growing pairs of horns that are almost interlocked and whose end points approach each other. The initial steps of the construction can be visualized with your fingers. Move the thumb and forefinger of each of your hands close to one another, then grow a smaller thumb and forefinger on each of these, and continue this budding without limit!

For additional information, see The Möbius Strip.
Artist: Cameron Browne
Click image.

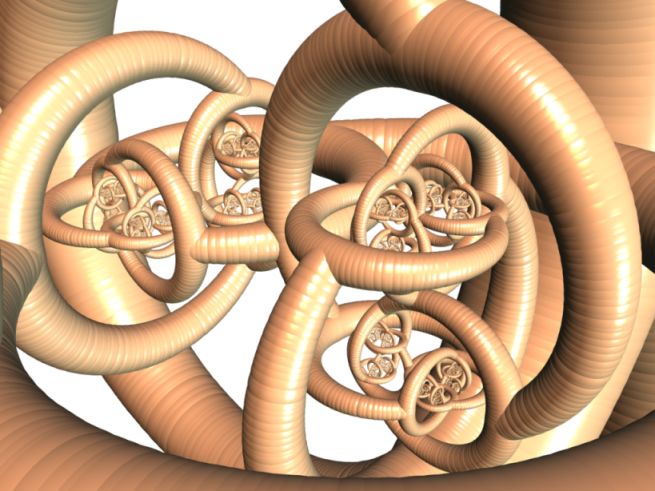
Although this may be hard to visualize, Alexander's horned sphere is homeomorphic to a ball. In this case, this means that it can be stretched into a ball without puncturing or breaking it. Perhaps it is easier to visualize the reverse: stretching the ball into the horned sphere without ripping it. The boundary is, therefore, homeomorphic to a sphere.
I show many more views of this object, provide additional information, and discuss the artist Cameron Browne in my book The Möbius Strip: Dr. August Möbius's Marvelous Band in Mathematics, Games, Literature, Art, Technology, and Cosmology.

Return to Reality Carnival.

If you like stories like this, Reality Carnival has many more.