On the sci.fractals newsgroup, Arne Dehli Halvorsen proposed a 3-D system of chaotic flows that are symmetric with respect to cyclic interchanges of x, y, and z. A large number of such systems were examined, and their symmetric attractors are available as 3-D anaglyphs.
One example of such a system is
dx/dt = - ax - 4y - 4z - y2
dy/dt = - ay - 4z - 4x - z2
dz/dt = - az - 4x - 4y - x2
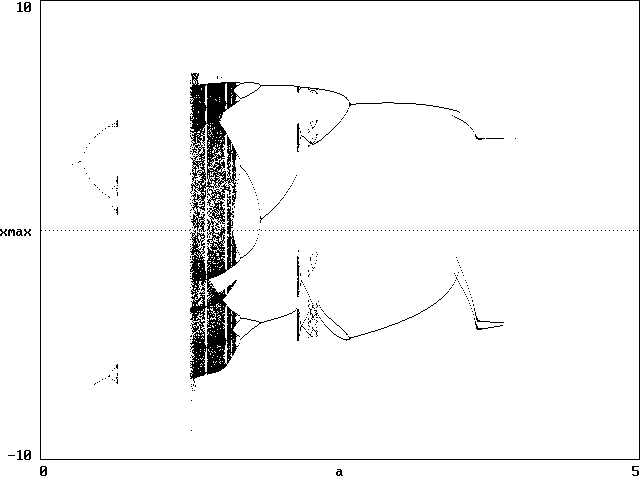
A bifurcation diagram of the above system, in which successive maximum values of x are plotted versus a, was calculated using the BASIC code SYMMETRY.BAS and compiled for DOS as SYMMETRY.EXE. The result is shown below:

The diagram shows a period-doubling route to chaos as a
is
decreased,
with a fully chaotic region at about a = 1.27. Eight
different
initial conditions chosen randomly over a cube with a sides of
length
10
centered on the origin were used for each value of a.
There
appear
to be multiple basins of attraction for some values of a.
Below
is an anaglyphic view of the attractor for a = 1.27 (use
red/blue
glasses)  :
:

Recently, Rene Thomas in Int. J. Bifurcation and Chaos 9, 1889-1905 (1999) proposed an even simpler class of system that possesses the same symmetry:
dx/dt = - ax + f(y)
dy/dt = - ay + f(z)
dz/dt = - az + f(x)
where f is one of a variety of nonlinear functions. The conservative limit of this sytstem with a = 0 also has chaotic solutions. A particularly interesting and elegant case is the one with f(x) = sin(x), which has an infinite number of fixed points arranged on a 3-D lattice. The chaotic trajectory percolates within this lattice of unstable steady states. He calls this "Labyrinth Chaos".
Both of these systems, and many more, are described in J.
C. Sprott, Chaos and Time-Series Analysis, Oxford
University
Press
(2003) and in J.
C. Sprott, Elegant Chaos, World Scientific (2010).